
| The Architecture of Curved Shapes [1] |
|---|
Cracow University of Technology
Department of Architectural Design
Cracow, Poland
INTRODUCTION
We
can analyse the architecture of a building in many different
ways. Let us name three main categories:
- formal analysis
- functional analysis
- structural analysis.
In my opinion, formal analysis is the most important because it can show us a thin line that divides architecture from construction (or, the construction industry), and it can also show us architecture as an art.
In the 20th century, architecture remains the part of art
where formal principles are very important for creators and spectators.
Architectural critics are still around and have something to
say because formal aspects of architecture can be criticised.
Because form in architecture is so important, two questions arise.
How can architects nowadays create forms? How can forms be described
and classified?
When we work only with formal
analysis, we can point to an important criterion of innovation,
that is, certain forms have never before been seen in the history
of architecture.
I.M. Pei's Pyramids at the Louvre don't belong to this category,
because he used very well known forms; only their locations within
the urban structure and the use of materials is quite new. But
I find the latest works of Frank Gehry and Peter Eisemann very
close to my idea of new forms in architecture at the end of the
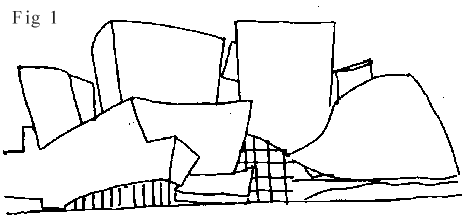
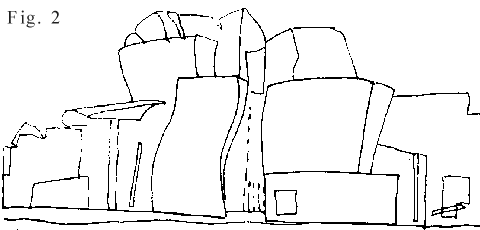
century. The Guggenheim Museum in Bilbao (Figure 1) and the Disney
Centre Hall (Figure 2), both designed by Gehry, display new ways
of creating architecture very similar to those described as curved-line
architecture by Chwistek in his theoretical works in the early
years of the 20th century.[2]
Is it a coincidence that forms of this kind emerged
at the end of millennium, at a time when information technology
has such a strong influence on our lives? For me, this is a rhetorical
question. It is absolutely clear that the imagination of architects
has never been constrained by classical geometry, but the fact
is that at the end of the 20th century, perhaps for the first
time, we can see material signs of this thinking in constructed
buildings.
I would like to make two important
points about the relation between the conventions of computer
modelling and the creation of architectural forms
[3] and the influences of CAD/CAM
(CAD, Computer Aided Design; CAM, Computer Aided Manufacturing) technology, which I call practical geometry,
in construction of a curved shapes building. CAD programs today
use three main conventions of representing space:
- Constructive Solid Geometry (CSG)
- Boundary Representation
- Distribution Functions (Methagraphics)
CONSTRUCTIVE SOLID GEOMETRY
When we have seen Roman Temples, we can
easily describe their geometry by algebraic Functions such as
addition, subtraction and multi plication of primitives (in this
case, primitives are cylinders, cubes, globes, etc).
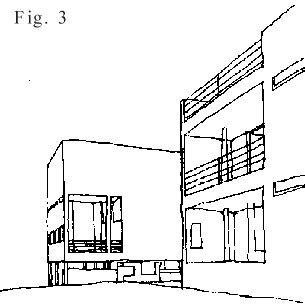
It is in this same way that CAD programs work in CSG mode (convention). Modern architecture, on the one hand, and traditional architecture, on the other, give us very good formal examples of such kind of space description. Buildings designed by Frank Lloyd Wright and Richard Meier (Figure 3) are typical example of this convention.
BOUNDARY REPRESENTATION
When
we see the amazing play of surfaces slashing at space, very characteristic
of the deconstructivist movement in architecture, we can clearly
see what boundary representation means in CAD space description.
In this method a solid is defined by determining
all points lying on its surfaces. In practice this is done by
the use of triangles, or analytical functions such as Beziers.
We can say that very similar forms for this type of CAD space
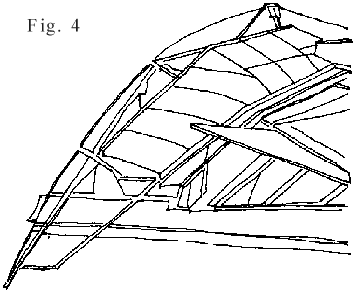
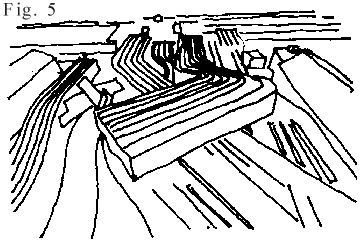
description are those designed by Zahah Hadid (with the exception
of her latest design for the Museum of Contemporary Art in Rome,
Figure 5) and the architecture group called Coop Himmelblau (Figure
4).
DISTRIBUTION FUNCTIONS
Distribution Functions is the last
method of space description used in CAD programs, and is especially
suitable for creating architecture of curved shapes. Where in
the former method several thousand polygons would be necessary,
here only a few dozen mataelipsoides are needed.
Some
projects of Frank Gehry (Figure 2), Peter Eisemann and the latest
design of Zahah Hadid (Figure 5) are good formal examples of
such kind of Space description used in CAD programs.
Methagraphics is especially interesting for us in the case of
the creation of curved architecture. Methagraphics will describe
such a form such as Gehry's design of Disney Centre Hall, but
more importantly, here was the possibility of using CAD/CAM technology
in the construction of a curved stone curtain wall. In this case,
they used Catia software, well known in the space shuttle construction.
CONCLUSION
Finally, at the end of the
20th, we can be proud to have the ability to create new forms
in architecture, both in our minds and in reality, made possible
by computers and geometry.
NOTES
[1]Leon Chwistek , Polish
mathematician and painter, 1884-1944, first defined the term
"Architecture of curved shapes" in his theoretical
design based on the Krywañ mountain in the Tatras.
return to text
[2] See Kazimierz Pasenkiewicz, Selected works of Leon Chwistek,vol.1 (Warsaw, 1961), vol.2 (Warsaw, 1963). return to text
[3] K. Butelski,
"On Similarites Between The Conventions of Computer Modeling
and The Creation of Architectural Form", ECAADE (Education
in Computer Aided Architectural Design Europe) XIII International
Conference, "Multimedia and Architectural Disciplines",
Dipartamento di Progetto e Construzione Edilizia, Università
di Palermo, 16-18 November 1995, pp. 71-74. return
to text
RELATED SITES ON THE WORLD WIDE WEB
KAZIMIERZ BUTELSKI'S SITES
Homepage
Virtual Design StudioZAHA HADID
Skewarch.com: Zaha Hadid
Zaha Hadid
Zaha Hadid painted projectsPETER EISENMAN
Skewarch.com: Peter Eisenman
Peter Eisenman biographyFRANK GEHRY
Skewarch.com: Frank Gehry
Frank Gehry image tour
The Walt Disney Concert Hall
Guggenheim Museum, BilbaoCOOP HIMMELBLAU
Skewarch.com: Coop Himmelblau
Great Buildings Online: Office Extension in Vienna
ABOUT
THE AUTHOR
Architect
Kazimierz Butelski was born in Cracow, Poland. He studied architecture
at Cracow University of Technology from 1983-1989. He studied
in the US in 1987, and was science assistant to Prof. Wojciech
Buli?ski at Cracow University of Technology faculty of Architectural
Design in 1989. He studied in France in 1991, and in Russia (Moscow,
Petersburg) in 1992. He is currently working on his doctorate
on the principles of planning and design methodology in the context
of CAD. He has worked with architect Romuald Loegler of Loegler
& Partnership (1988-1989) , Prof. Arch. Zbigniew Kupiec (1989-1991),
PUA Ltd (1992-present), and has co-operated with architect Janusz
Purski BP Project Ltd on projects, theoretical works, awards
and competitions. In 1994 he received the Cracow Scholarship
in Architecture for Young Artist. He lives and works in Cracow.
