
| The Double Möbius Strip Studies |
|---|

|
Assistant Lecturer, Faculty of Architecture University of Ljubljana, Slovenia Art Director, Arxel Tribe Ljubljana, Slovenia |
INTRODUCTION
In
the eighteenth century, Euler (1707-1783) observed that the solids
enclosed by plane faces have the number of vertices (V) minus
the number of edges (E) plus the number of faces (F) that equals
2, the formula therefore being V - E + F = 2. But this formula
does not work for all polyhedra -- e.g., a polyhedron with a
hole -- so the constitution of a hole in a solid as well as its
relation to Euler's formula for polyhedra was questioned.
According to Peterson [1], the astronomer and mathematician August Ferdinand Möbius (1790-1868) studied the development of a geometrical theory of polyhedra and identified surfaces in terms of flat polygonal pieces variously glued together. The curious single continuous surface named after him has only one side and one edge, and is made by twisting the band by 180° then joining the two ends. When following the path of its surface, one can reach any other point without ever crossing an edge. The phenomenal one-sidedness of the band can be explained if its surface is divided into a row of triangles and subsequently twisted and joined at its ends. Möbius published his discoveries in 1865 in his paper "On the Determination of the Volume of a Polyhedron", also revealing that there are polyhedra to which no volume can be assigned. But it was only in the past century that attention in mathematics was drawn to studies of hyper- and fractal dimensions.
THE CURIOUS BAND BETWEEN DIMENSIONS
The Möbius strip is a mathematical construction
demonstrating an evolution of a two-dimensional plane into a
three-dimensional space; by merging the inner with the outer
surface, it creates a single continuously curved surface. It
allows returning to the point of departure after having completed
a tour by following a path along its surface. This paradox can
be explained by the fact that even though the strip has only
one side, each point corresponds to two sides of its surface.
Similarly, the Weierstras curve does not have one single tangent
even though it is a continuous geometrical formation.
Another interesting situation appears when a band with joined ends is cut in half lengthwise until getting back to the starting point: a single band twice as long as the original is produced if its ends have been rotated for 180°, but rotating its ends for 360° forms two interlocking rings. Yet the Möbius strip is far more than just a mathematical abstraction. Symbolically, its two-dimensional projection forming the figure eight represents infinity and cycles, but can also be found in many natural phenomena related to fluid dynamics and the analemma. The latter is known as a representation of the virtual path of the sun projected to the surface of the Earth. It reveals the dynamics of sunlight as a source of our vision and an instrument of construction of our space-time perception. Representing temporality, the cyclical nature of processes and eternity, it is no wonder that the twisted ring is an archetype, a symbol of infinity, present both in alchemistic iconography as the serpent biting its tail, named the ouroboros and in contemporary consumer society as an icon of recycling. Since its discovery in nineteenth century, the Möbius strip has been largely used not only in science, engineering, music, literature and art, but also as a means of exploring the synergy of geometry, movement and sound [2].
THE FIGURE EIGHT AS A SYMBOL
Balmond [3]
uses the figure eight, also used as the symbol of infinity in
mathematics, to graph relations between the numbers 5 and 4,
6 and 3, 7 and 2, 8 and 1 in an asymmetrical way. Recent studies
of analemma which also mimics the figure eight show that its
asymmetry is a result of the sun being projected to the curved
surface of the Earth, but purging this deformation [4] produces a symmetrical figure eight.
In Balmond's work, numbers between one and eight are paired as
twins in multiplication tables: in this way, number nine is the
sum of numbers 5 and 4, 6 and 3, 7 and 2, 8 and 1; presented
in the sigma code (the sum of digits of a number), the pairs
of numbers will always sum up to 9 even if both of the twins
have been multiplied. These relations can be graphed in the form
of a figure eight or a Möbius strip: when such a graphed
numeric orbit flows through the inflection point (number 9) in
a clockwise sense, it subsequently reverses itself into its own
twin partner, continuing the flow through an anticlockwise orbit.
Balmond explains: "Quite unlike the stationary circles,
energy is released into the numbers so that they spin, one out
of the other … the bending and twisting in and out of separate
energies, the big and the small, connected by a continuous movement
through the eye at the centre of the storm of numbers."
[5]
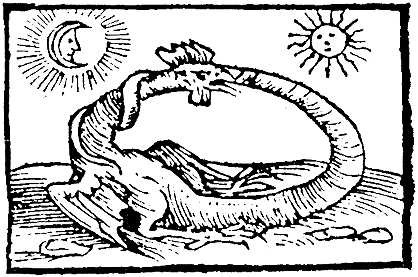 Symbolically,
the eternal revolving is traditionally symbolised by the ouroboros, representing a continuous
circle of creation. According to Cusanus, the circumference completes
the centre to suggest the idea of God. Being a symbol of the
manifestation and cycles, the ouroboros
represents unity, self-nourishment, union of matter and spirit;
in hermetic tradition, it symbolises the union of Isis and Osiris
- the Female and the Male principle represented also by two intertwining
serpents related to the Sun and the Moon; as such, it has also
been extensively used in Alberti's architecture. It symbolises
a dialectics of life and death, the dynamics of circle, infinite
movement, universal animation and is therefore extremely interesting
as a subject of research in architectural animation. The ouroboros is a creator of time, duration
and life and continuously returns to itself. The alchemists'
Big Whole is a cosmic spirit, a symbol of eternity and cyclic
time, also used by Cusanus as a symbol of Divinity [6]. An outstanding parallel can be drawn
to the Zen tradition, based on the dynamic sphere of the two
opposite principles in a perpetual interaction, the Yin and the
Yang.
Symbolically,
the eternal revolving is traditionally symbolised by the ouroboros, representing a continuous
circle of creation. According to Cusanus, the circumference completes
the centre to suggest the idea of God. Being a symbol of the
manifestation and cycles, the ouroboros
represents unity, self-nourishment, union of matter and spirit;
in hermetic tradition, it symbolises the union of Isis and Osiris
- the Female and the Male principle represented also by two intertwining
serpents related to the Sun and the Moon; as such, it has also
been extensively used in Alberti's architecture. It symbolises
a dialectics of life and death, the dynamics of circle, infinite
movement, universal animation and is therefore extremely interesting
as a subject of research in architectural animation. The ouroboros is a creator of time, duration
and life and continuously returns to itself. The alchemists'
Big Whole is a cosmic spirit, a symbol of eternity and cyclic
time, also used by Cusanus as a symbol of Divinity [6]. An outstanding parallel can be drawn
to the Zen tradition, based on the dynamic sphere of the two
opposite principles in a perpetual interaction, the Yin and the
Yang.
THE MÖBIUS STRIP IN ARCHITECTURE
The Möbius strip has a great potential as an architectural
form, but we can also use its dynamics to reveal the mechanisms
of our perception (or rather, its deceptions as in the case of
optical illusions) in an augmented space-time. According to Baldino
and Cabral [7],
Lacan used to invite subjects to articulate the psychoanalytical
discourse by resorting to logic and even to definition of compact
spaces by open coverings. He used topological formalisations
such as Möbius strip, Riemannian surfaces, Borromean knot
and Klein bottle to reveal the unconscious mechanisms responsible
for the psychic configuration of reality in order to weaken the
subject's faith in his stable permanent ego.
Hartmann [8] argues that the Möbius strip subverts the normal, i.e. Euclidean way of spatial and temporal representation, seemingly having two sides, but in fact having only one. At one point the two sides can be clearly distinguished, but when you traverse the strip as a whole, the two sides are experienced as being continuous. Lacan also employs the Möbius strip as a mode to conceptualise the "return of the repressed" as well as to illustrate the way psychoanalysis reconceptualises certain binary oppositions (inside/outside, before/after, signifier/signified, etc.).
In Lacanian terminology, it is by suturing off the real that the reality of the subject remains a coherent illusion that prevents him or her from falling prey to the real. Similarly, reversible images that have first been studied in Gestalt psychology and explored by many artists, carry a double meaning, their significance shifting from one figure to the other in loops just like moving through a Möbius strip topology. If reversible images are said to demonstrate the dynamics of human visual perception and visual thinking by opening a two-dimensional surface into a three-dimensional space, introducing time into spatial dimensions, a similar principle could be identified in Möbius strip: it exists between dimensions, i.e. in fractal dimensions. Introducing topology into architectural space, its curious geometry has made architects rediscover the Möbius strip in the age of information society, topological architecture and hypersurfaces.
As a consequence of the paradigmatic shift, generated by the electronic revolution, both science and culture began to question their Cartesian foundations. As a result of the introduction of non-linear dynamics, relativity, indeterminacy and topological geometry, architecture started to reflect dynamics and animation as a cultural condition rather than the principle of stasis, establishing a new formal vocabulary. Within the physical sciences, topology theory is regarded as the rubbermath or rubber sheet geometry. It is also a conceptual and qualitative paradigm - deforming shapes without destroying their essential properties. Topology theory entails deformation processes such as pulling, twisting, stretching, turning and contorting, but no configuration of shapes using cutting, tearing or pasting.
The idea of a structure containing a loop appears in projects for a Möbius House by Stephen Perrella, by Architectonics or by UN Studio and the Möbius strip design at Laguna Beach by Perrella, Robert and Petresin. Here, the Möbius strip is used as a diagram for post-Cartesian dwelling and is neither an interior space nor an exterior form: it is a transversal membrane reconfiguring these binary notions into a continuous, non-linear form, thus creating a basis of a complex, temporal experience. It is interesting how the dynamics of dwelling can be described as a cyclical activity or a process in the interior and exterior space, tied to fixed trajectories on a torus. Attractors in a vertical and a horizontal direction can move in loops or figures of eight around such a torus. By analogy, the dualities in contemporary dwelling, e.g. the concepts of inside/outside, below/above, physical/virtual, private/non-private, material/media can be represented as such attractors; these form twisted tracks as on a Möbius strip where one end is twisted and then joined to the other end to create a single continuous surface.
The Möbius diagram adapts a metaphorical looping and by its conversion of binary notions in architecture, it offers new possibilities for architecture where the inside of the object becomes the outside; where the media penetrates the private sphere, the unique is tied to the general through a trajectory of this virtual form, where floor become walls become floor or where a place is reversed into a non-place. This is why the Moebius strip has become a geometrical structure par excellence of topological architecture, being a relatively adequate representation of dwelling activities.
DIGITAL MODELING OF A MÖBIUS STRIP
Studying the Möbius strip is a complicated task when using
a physical model, but the current technology enables us to model
and reconfigure it in a digital environment. For the following
studies, the software Maya Unlimited 4.0 (Alias/Wavefront) was
used.
In order to model a surface, a minimum of two curves are needed to define its edges; the problem of the Möbius strip is that its single continuous surface only involves one curve along the band's edges in a Cartesian space - a paradox that discontinues processes in the modeling programme. A solution would therefore be to use a minimum of two curves: let us cut the Möbius curve into two isolated curves and reintegrate them so the computer can recognise them as a composed object, thus enabling its calculation and building a surface. A regular surface being a flat, two-dimensional construction in a Cartesian space, its normals are perpendicular to its surface having a common orientation (positive or negative). A digitally constructed surface is divided into faces, their direction being defined by their normals. A surface can only be closed when the normals of its two extreme faces have identical orientation. But the surface of a Möbius strip that we tried to create by lofting the composite curve resulted in a paradoxical formation: the normals of the faces at the adjoining edges of the band have opposite direction that makes it impossible to join the band's borders thus closing the surface. Modeling an infinitely continuous surface in a virtual environment that is limited to being an open-ended structure means that it can not be recreated in a Cartesian space.
Assigning a curve having a singular orientation to the surface of the Möbius strip to create a volume (i.e. a corridor) results in a similar paradox: if the curved profile of the structure following the path of the Möbius surface has positive direction at the starting point of the translation, it will have a negative direction after having completed the path and revolving to the starting point. Obviously, studying a form that exists between the second and the third dimension relates to a fractal quality, but is there a way of taming the computer one more time?
Instead of using a single profile, the curve can be copied, applied at the scale of -1 and translated along the path of the Möbius surface to define a new volume. The section of this new structure contains rotational or mirror symmetry regarding the normals of the Möbius strip faces, meaning that its adjoining edges can be glued together thus forming a single continuous tubular volume or a warped torus.
INTRODUCING THE DOUBLE MÖBIUS STRIP
A Möbius strip has now been successfully reconfigured as
a volume in a virtual environment using the software Maya 4.0,
but let us go back to the problem of modeling a Möbius surface:
this can be rendered as a continuous topological entity if the
simple band with a continuous (yet still a composite) surface
is duplicated and consequently transformed by applying a negative
scale (x = -1, y = -1). This inversion results in a closed, continuous
structure that we called the Double Möbius Strip. The same
process can be applied to a simple twisted band describing a
volume.
The fractal geometry of the double Möbius strip becomes evident by identifying its characteristics as applied to architectural space. If the two intertwining bands of the double Möbius strip represent the structure of a wall and a floor, the two architectural articulations can become mutually entangled and exchanging while following a path along the two surfaces. Starting on a horizontal surface representing the floor results in moving along the surface of the wall become floor after having completed a tour and vice versa. It is also interesting to notice that entering the structure from a particular band always results in revolving to the starting point of the identical band. If the double Möbius structure is transparent, its users can walk along the paths of both strips representing floor-become walls-become floor and never interfere with the users starting from a different entrance placed at the other strip as the surfaces never intersect (Figure 1).
These interesting characteristics do not only indicate the non-Euclidean nature of the double Möbius strip, thus making it an ideal polygon for formal and conceptual research in post-Cartesian architecture, they also refer to a possibility of reversing and even uniting archetypal binary notions of surface/volume, space/time, inside/outside, matter/media etc. In architecture based on topological geometry, the traditional articulation of surfaces forming ceilings, walls and floors is absent as the structural members known in classical composition melt into a fluid, dynamic entity.
Let us step back and consider another interesting analogy that can be established between the double Möbius strip, its principle of unity through perpetual duality, and the DNA (discovered by Watson, Crick and Wilkins): the model of the double helix is composed of two serpent-like intertwining spirals, representing a biological reflection of the archetypal idea of time as a spiral, creating a reunion of the linear and the cyclical aspects of time as a perpetual flow. The idea of unity through continuity also appears as the most important characteristic trait of structure in Lacan's lecture on Structure & Reality.[9] If the previous Gestalt notion of good form has been related to its function of joining and producing the "unifying unity", Lacan on the other hand reconsiders good form as a "countable unity" one, two, three etc., as an integer that can be used for counting with the formula (n + 1). In this way, the question of "one more" actually becomes the key to the genesis of numbers and has been applied in our attempt to generate the double Möbius strip.
RECONFIGURING THE DOUBLE MÖBIUS STRIP
Let us briefly indicate some of the possibilities of reconfiguring
the surfaces created by the dynamics of the double Möbius
strip in an architectural context. Another simulation has been
made to explore possible architectural constructions. The two
Möbius bands have been structurally interconnected to create
a continuous spatial entity. In order to structure the membrane
following the pathways of the strips, the surface can be divided
as it was initially shown by some of the Möbius' own studies
in triangulation of the twisted bands as mentioned above in the
text. Using non-uniform rational B-splines (NURBS) to model the
strips, the structure is manipulated by transformable grids,
but to give them a minimal operational surface shape, it can
be assigned triangles as polygons containing the least edges
and vertices; in this way, a system of subdivision of the surface
can be applied to obtain a triangulation on a previously square-faceted
surface. If the system is applied twice and maintained planar,
an optical illusion revealing a structure of hyper-solids is
achieved (Figure 2).
Similarities in form and movement of the double Möbius strip structure can be established with the structure and the nature of formation of a vortex. This fractal phenomenon of fluid dynamics has brought forward a connection with our previous research in applications of processes of particle physics to create and control deformations (Figure 3). In the next step, a double Möbius strip has been used to study the behaviour of a system of forms exposed to nature-like processes. The dynamic and the process-based contemporary cosmology has introduced the principles of transformation and deformation as an architectural condition. Non-Euclidean and non-linear geometry, indeterminism, relativity and quantum physics can be reflected in architectural form generation as well as the dynamics of transformation processes through deformation. In a responsive environment, particle physics can be used to deform surfaces.
TRANSFORMATION OF THE DOUBLE MÖBIUS
STRUCTURE USING PARTICLE PHYSICS
In
3D modeling, reactions of the construction points of a surface
or a volume in the action field can be controlled individually
and exposed to various processes of deformation such as simulation
of mathematical and physical phenomena or natural algorithms.
This can be achieved by defining the construction points of the
chosen object as particles with proper identities. Processes
of deformation can be applied in an unlimited duration, demonstrating
the dimension of time as the agent in the generation of architectural
/ animated forms.
Using the principles of particle physics in animation software allows architectural composition to exceed its Cartesian limitations as well as enable extremely complex topological architecture a quantifiable descent into the built environment. In fact, topological surface-based forms bring forward the issue of mathematically determined space, be it Euclidean or Riemannian, and its relation to organic form.
Cartesian and Euclidean notions of space seem to be no longer accurate to describe topological architecture. Classical architectural composition relies on mathematical tools to create or analyse the generation of form. In a computer generated architectural environment, the language of mathematics can once more become a powerful instrument of non-linear composition and a way to avoid purely random search for beautiful images; forces within a field can be programmed to simulate processes known from fluid dynamics and particle physics (Animation 1).
Dynamic systems theory mainly relates to non-linear processes in motion and their behaviour. Such systems are known to have inseparable space-time, a process-dependent, contingent observer and non-local causality. Fields that give an input to the system function as complex, non-linear, multidimensional networks, where every particular action is linked to that of another entity and every moment is irreversible. They are composed of delocalised entities, i.e. the particles with mutually entangled space-times. The particles are dynamic, fluid and can change in the course of development either as a result of feedback or directly as a response to the environment; these changes influence subsequent behaviour of the system. It is obvious that such premises are highly relevant for a creation of a responsive (interactive) environment with an entanglement of the observer and the observed. Our research was oriented towards operations in which the object (or a particle within a field) is immersed as agent within general conditions of creating forms, processes, and fluxes.
Processes of metamorphosis such as the transformation, the dynamic principle in which destruction of a particular state is necessary in order to proceed discretely to the next level or a different form of manifestation have been the key elements of our research. In animation and creation of special effects, principles of particle physics are integrated within the software to simulate natural phenomena, but it is also possible to use them to demonstrate particular processes and their effects in a virtual environment as applied to the structure of a double Möbius strip. The reactions of the construction points of a surface or a volume within a field can be controlled and traced individually as well as exposed to various physical processes. Fields are forces used to animate the motion of particles. We have defined every construction point or vertex of a surface as a particle with its own identity and characteristics, subsequently exposing them to processes of physical and mathematical force fields (Animation 2).
In a zero frame (0,0), proper identities can be defined for each particle and their relative force fields. We have not been operating from a point of view of a surface deformation, but from singular dimensionless entities activated in a space-time system that allow its evolution. A series of independent movements causing system transformations within the time line has been created (Animation 3). In our computer simulation, coordinate points of a NURBS primitive can be identified as particles that have a possibility of vector activity along the three axes. Every particle can be defined by expressions; the entire system can grow in time, accumulate, diminish or sum up deformations. When field activities can be applied and particles can be given attributes such as magnitude, attenuation, frequency, maximum distance, phase and noise level/ratio. Animation of every single vertex of a surface results in a deformation of the body (Figure 4 and Figure 5).
In physical architectural space, mechanical and electronic systems such as joints and sensors can be applied to structures to create a kinetic architecture to simulate particles and field activities. A great example of a computer generated process translated to a built structure is the Aegis Hyposurface by dECOi (10). The kinetic potential of inert architectural form can be studied using animation software and motion capture.
The studies in Möbius strip raise further questions on our perception of space and the mechanisms of optical illusions, but they have also been aimed at re-establishing the central role of the observer/user as known from the relativity theory, thus integrating the human scale within a culturally and technologically rapidly evolving environment. Human activities could be monitored as force fields creating an input to the space and its meaning. Therefore the role of the observer/user adapting to his environment can be compared to the idea of developing intelligent spaces interacting with their users.
REFERENCES
[1]
I. Peterson, Möbius
and his Band, Science News Online, 8. 8. 2000, www.sciencenews.org/20000708/mathtrek.asp.
return to text
[2] G. Jobin and F. Treichler. The Möbius Strip, Theatre Aux Abbesses, Paris 2001. return to text
[3] Cecil Balmond, Number 9, The Search for the Sigma Code. Munich, New York: Prestel, 1998. return to text
[4] E. Petresin, Hypothesis of Fluid Dynamics / Hipoteza gibanja tekocin. University of Maribor, 2001. return to text
[5] Balmond, ibid., 130. return to text
[6] Nicholas of Cusa, Idiota da sapientia. See also: W. Schultze, Zahl, Proportion, Analogie (Muenster: Aschendorff, 1978). return to text
[7] R.R. Baldino and T.C.B. Cabral, "Lacan's Four Discourses in Mathematics Educational Credit System", http://s13a.math.aca.mmu.ac.uk/Chreods/Issue_13/4Discourses. return to text
[8] Bernd Herzogenrath, "Digression 2: How to Make a Moebius Strip", in "On the Lost Highway: Lynch and Lacan, Cinema and Cultural Pathology." Other Voices, vol.1, no. 3 (January 1999). return to text
[9] J. Lacan, "On Structure & Reality" (lecture), http://lacan.com/hotel.htm. return to text
[10] dECOi: Aegis Hyposurface, Birmingham Hippodrome Foyer Artwork Competition (1st prize), 1999.
RELATED SITES
ON THE WWW
Strolling
Down Möbius Lane
ABOUT THE AUTHORS
Vesna
Petresin is an architect based in London. After having studied
at the Musical Conservatory in Ljubljana and having worked as
an artist/designer, she went to teach at the Faculty of Architecture
in Ljubljana, also collaborating with the Architectural Association,
the RMIT, Paris-la-Villette and Oxford Brookes. She obtained
her Ph. D. in temporal aspects of architectural composition.
She taught design studios with Mark Goulthorpe (decoi)and
Tom Kovac and has worked for Stephen Perrella's Hypersurface
Systems. With Laurent-Paul Robert, they formed Rubedo in 2001,
and have published and exhibited internationally. Her current
research interests include visual perception and design theories,
as well as the impact of emergent technologies on architecture.
Laurent-Paul Robert is a French/Swiss artist and computer animator based in London. After having worked as an artist, performer and musician, he has continued in 3D animation since 1994, becoming the lead art director and senior animator for Arxel Tribe. He also worked in architecture with Stephen Perrella's Hypersurface Systems, and has continued his design and research with Vesna Petresin as Rubedo since 2001; he has exhibited and published internationally. He is currently working as a consultant for clients such as Arup and decoi.
|
Vesna Petresin and Laurent-Paul Robert, "The Double Möbius Strip Studies", Nexus Network Journal, vol. 4, no. 4 (Autumn 2002), http://www.nexusjournal.com/PetRob.html |
Copyright ©2002 Kim Williams Books

