7 Mixed Geometry: Einstein’s New Attempt
After his move to Princeton, Einstein followed quite a few interests different from his later work on UFT. In the second half of the 1930s he investigated equations of motion of point particles in the gravitational field in the framework of his general relativity theory (with N. Rosen L. Infeld, and B. Hoffmann), and the conceptual intricacies of quantum mechanics (with B. Podolsky and N. Rosen). As we have seen in Section 3.2, in 1938 – 1943 he had turned back to Kaluza’s 5-dimensional UFT (with P. G. Bergmann, V. Bargmann, and in one paper with W. Pauli) to which theory he had given his attention previously, in 1926 – 1928; cf. Section 6.3 of Part I. After joint work with V. Bargmann on bi-vector (bilocal) fields in 1944 (cf. Section 3.3.1), he took up afresh his ideas on mixed geometry of 1925. He then stayed within this geometrical approach to UFT until the end of his life.In the period 1923 – 1933 Einstein had tried one geometry after the other for the construction of UFT, i.e., Eddington’s affine, Cartan’s tele-parallel, Kaluza’s 5 dimensional Riemannian geometry, and finally mixed geometry, a blend of affine geometry and Foerster’s (alias Bach’s) idea of using a metric with a skew-symmetric part. Unlike this, after the second world war he stuck to one and the same geometry with asymmetric fundamental tensor and asymmetric affine connection. The problems dealt with by him then were technical at first: what fundamental variables to chose, what “natural” field equations to take, and how to derive these in a satisfactory manner. Next, would the equations chosen be able to provide a set of solutions large enough for physics? Would they admit exact solutions without singularities? In physics, his central interest was directed towards the possibilities for the interpretation of geometrical objects as physical observables. During his life, he believed that the corpus of UFT had not yet become mature enough to allow for a comparison with experiment/observation. His epistemological credo lead him to distrust the probability interpretation of quantum theory as a secure foundation of fundamental physical theory; for him quantum mechanics amounted to no more than a useful “model”. His philosophical position may also have demotivated him to the extent that, already in the late 1930s, he had given up on learning the formalism of quantum field theory in order to be able to follow its further development.88 To see him acquire a working knowledge of quantum field theory as a beginner, after World War II, would have been asking too much in view of his age and state of health. That he did not take into account progress in nuclear and elementary particle physics reached in the two decades since he first had looked at mixed geometry, was a further factor isolating him from many of his well known colleagues in theoretical physics.
As we shall note in Section 10.5.1, in 1942 – 1944 Einstein’s interest was also directed to the question to what extent singularities occur in the solutions of the field equations of general relativity and of Kaluza–Klein. It is during this time that he wrote to Hans Mühsam in spring of 1942 (as quoted by Seelig [570*], p. 412):
“But in my work I am more fanatical than ever, and really hope to have solved my old
problem of the unity of the physical field. Somehow, however, it is like with an airship with
which we can sail through the clouds but not clearly see how to land in reality, i.e on the
earth.” 89
Einstein’s first three papers on UFT via mixed geometry ([142*, 147*, 179*]) all employ the metric 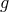 and the connection
and the connection 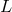 as independent variables - with altogether 80 available components in local
coordinates while just 6 + 10 of them are needed for a description of the gravitational and electromagnetic
fields. (The number of the inherent “degrees of freedom” is a more complicated affair.) A strategy followed
by Einstein and others seems to have been to remove the superfluous 64 variables in the affine connection
by expressing them by the components of the metric, its first derivatives, and the torsion tensor. Since the
matter variables were to be included in the geometry, at least in the approach to UFT by Einstein, enough
geometrical objects would have to be found in order to represent matter, e.g., 4 components of the electric
4-current, 4 components of the magnetic 4-current, 5 components for an ideal fluid, more for
the unspecified energy-momentum-tensor in total. In Einstein’s approach, the symmetric part
of the metric,
as independent variables - with altogether 80 available components in local
coordinates while just 6 + 10 of them are needed for a description of the gravitational and electromagnetic
fields. (The number of the inherent “degrees of freedom” is a more complicated affair.) A strategy followed
by Einstein and others seems to have been to remove the superfluous 64 variables in the affine connection
by expressing them by the components of the metric, its first derivatives, and the torsion tensor. Since the
matter variables were to be included in the geometry, at least in the approach to UFT by Einstein, enough
geometrical objects would have to be found in order to represent matter, e.g., 4 components of the electric
4-current, 4 components of the magnetic 4-current, 5 components for an ideal fluid, more for
the unspecified energy-momentum-tensor in total. In Einstein’s approach, the symmetric part
of the metric, 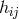 , is assumed to correspond to inertial and gravitational fields while the
antisymmetric part
, is assumed to correspond to inertial and gravitational fields while the
antisymmetric part 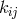 houses the electromagnetic field. The matter variables then are related to
derivatives of the metric and connection (cf. Section 10.3.1). The field equations would have to be
derived from such a Lagrangian in such a way that general relativity and Maxwell’s equations be
contained in UFT as limiting cases. Unlike the situation in general relativity, in metric-affine
geometry a two-parameter set of possible Lagrangians linear in the curvature tensor (with
the cosmological constant still to be added) does exist if homothetic curvature in (65*), (66*) of
Section 2.3.1 is included. Nevertheless, Einstein used a Lagrangian corresponding (more or less)
to the curvature scalar in Riemannian geometry
houses the electromagnetic field. The matter variables then are related to
derivatives of the metric and connection (cf. Section 10.3.1). The field equations would have to be
derived from such a Lagrangian in such a way that general relativity and Maxwell’s equations be
contained in UFT as limiting cases. Unlike the situation in general relativity, in metric-affine
geometry a two-parameter set of possible Lagrangians linear in the curvature tensor (with
the cosmological constant still to be added) does exist if homothetic curvature in (65*), (66*) of
Section 2.3.1 is included. Nevertheless, Einstein used a Lagrangian corresponding (more or less)
to the curvature scalar in Riemannian geometry 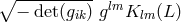 without further
justification.90
Such field equations, the main alternatives of which came to be named strong and weak, were used to
express the connection as a complicated functional of the metric and its derivatives and to determine the
two parts, symmetric and skew-symmetric, of the metric. This was fully achieved not before the 1950s;
cf. Section 10.1.
without further
justification.90
Such field equations, the main alternatives of which came to be named strong and weak, were used to
express the connection as a complicated functional of the metric and its derivatives and to determine the
two parts, symmetric and skew-symmetric, of the metric. This was fully achieved not before the 1950s;
cf. Section 10.1.
Interestingly, in his second paper of 1945 using mixed geometry, Einstein did not mention his first one of 1925. It seems unrealistic to assume that he had forgotten what he had done twenty years earlier. His papers had been published in the proceedings of the Prussian Academy of Science in Berlin. Possibly, he just did not want to refer to the Prussian Academy from which he had resigned in 1933, and then been thrown out. This is more convincing than anything else; he never ever mentioned his paper of 1925 in a publication after 1933 [312]. There is a small difference between Einstein’s first paper using mixed geometry [142] and his second [147*]: He now introduced complex-valued fields on real space-time in order to apply what he termed “Hermitian symmetry”; cf. (46*). After Pauli had observed that the theory could be developed without the independent variables being complex, in his next (third) paper Einstein used “Hermitian symmetry” in a generalized meaning, i.e., as transposition invariance [179*]; cf. Section 2.2.2.
7.1 Formal and physical motivation
Once he had chosen geometrical structures, as in mixed geometry, Einstein needed principles for
constraining his field equations. What he had called “the principle of general relativity”, i.e., the demand
that all physical equations be covariant under arbitrary coordinate transformations (“general covariance”),
became also one of the fundamentals of Einstein’s further generalization of general relativity. There, the
principle of covariance and the demand for differential equations of 2nd order (in the derivatives) for the
field variables had led to a unique Lagrangian 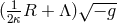 , with the cosmological constant
, with the cosmological constant  being the only free parameter. In UFT, with mixed geometry describing space-time, the situation was less
fortunate: From the curvature tensor, two independent scalar invariants could be formed. Moreover, if
torsion was used as a separate constructive element offered by this particular geometry, the arbitrariness in
the choice of a Lagrangian increased considerably. In principle, in place of the term with a single
cosmological constant
being the only free parameter. In UFT, with mixed geometry describing space-time, the situation was less
fortunate: From the curvature tensor, two independent scalar invariants could be formed. Moreover, if
torsion was used as a separate constructive element offered by this particular geometry, the arbitrariness in
the choice of a Lagrangian increased considerably. In principle, in place of the term with a single
cosmological constant  , a further term with two constants could be added:
, a further term with two constants could be added: 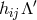 and
and
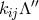 .
.
In his paper of 1945 Einstein gave two formal criteria as to when a theory could be called a “unified” field theory. The first was that “the field appear as a unified entity”, i.e., that it must not be decomposable into irreducible parts. The second was that “neither the field equations nor the Hamiltonian function can be expressed as the sum of several invariant parts, but are formally united entities”. He readily admitted that for the theory presented in the paper, the first criterion was not fulfilled ([147*], p. 578). As remarked above, the equations of general relativity as well as Maxwell’s equations ought to be contained within the field equations of UFT; by some sort of limiting process it should be possible to regain them. A further requirement was the inclusion of matter into geometry: some of the mathematical objects ought to be identified with physical quantities describing the material sources for the fields. With UFT being a field theory, the concept of “particle” had no place in it. Already in 1929, in his correspondence with Elie Cartan, Einstein had been firm on this point:
“Substance, in your sense means the existence of timelike lines of a special kind. This is the translation of the concept of particle to the case of a continuum. […] the necessity of such translatibility, seems totally unreasonable as a theoretical demand. To realize the essential point of atomic thought on the level of a continuum theory, it is sufficient to have a field of high intensity in a spatially small region which, with respect to its “timelike” evolution, satisfies certain conservation laws […].” ([116*], p. 95.)91
Thus, the discussion of “equations of motion” would have to use the concept of thin timelike tubes, and integrals over their surface, or some other technique. Einstein kept adamantly to this negatory position concerning point particles as shown by the following quote from his paper with N. Rosen of 1935 [178]:
“[…] writers have occasionally noted the possibility that material particles might be considered as singularities of the field. This point of view, however, we cannot accept at all. For a singularity brings so much arbitrariness into the theory that it actually nullifies its laws.”
As we will see below, an idea tried by Einstein for the reduction of constructive possibilities, amounted
to the introduction of additional symmetries like invariance with respect to Hermitian (transposition-)
substitution, and later, 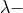 transformations; cf. Sections 2.2.2 and 2.2.3. Further comments on these
transformations are given in Section 9.8.
transformations; cf. Sections 2.2.2 and 2.2.3. Further comments on these
transformations are given in Section 9.8.
Interestingly, the limiting subcase in which the symmetric part of the (asymmetric) metric is assumed to be Minkowskian and which would have lead to a generalization of Maxwell’s theory apparently has been studied rarely as an exact, if only heavily overdetermined theory; cf. however [450, 600, 502*].
7.2 Einstein 1945
As early as in 1942, in his attempts at unifying the gravitational and electromagnetic fields, Einstein had considered using both a complex valued tensor field and a 4-dimensional complex space as a new framework. About this, he reported to his friend M. Besso in August 1942 ([163*], p. 367):
“What I now do will seem a bit crazy to you, and perhaps it is crazy. […] I consider a space
the 4 coordinates 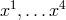 which are complex such that in fact it is an 8-dimensional
space. To each coordinate
which are complex such that in fact it is an 8-dimensional
space. To each coordinate 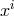 belongs its complex conjugate
belongs its complex conjugate 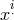 . […] In place of the
Riemannian metric another one of the form
. […] In place of the
Riemannian metric another one of the form 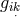 obtains. We ask it to be real, i.e.,
obtains. We ask it to be real, i.e.,
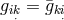 must hold (Hermitian metric). The
must hold (Hermitian metric). The  are analytical functions of the
are analytical functions of the 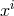 ,
and
,
and 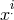 . […]”92
. […]”92
He then asked for field equations and for complex coordinate transformations. “The problem is that there exist several equations fulfilling these conditions. However, I found out that that this difficulty goes away if attacked correctly, and that one can proceed almost as with Riemann” ([163*], p. 367–368).
During the 3 years until he published his next paper in the framework of mixed geometry, Einstein had changed his mind: he stuck to real space-time and only took the field variables to be complex [147*]. He was not the first to dabble in such a mathematical structure. More than a decade before, advised by A. Eddington, Hsin P. Soh , during his stay at the Massachusetts Institute of Technology, had published a paper on a theory of gravitation and electromagnetism within complex four-dimensional Riemannian geometry with real coordinates. The real part of the metric “[…] is associated with mass (gravitation) and the imaginary part with charge (electromagnetism)” ([581], p. 299). Einstein derived the field equations from the Lagrangian93
with94 and Here,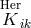 is the Hermitian-symmetrized Ricci-tensor obtained from the curvature tensor (54*) as:
is the Hermitian-symmetrized Ricci-tensor obtained from the curvature tensor (54*) as:
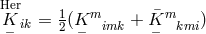 (cf. (73*) of Section 2.3.2):
with
(cf. (73*) of Section 2.3.2):
with 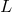 being a connection with Hermitian symmetry. Note that
being a connection with Hermitian symmetry. Note that 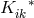 is not Hermitian thus implying a
non-Hermitian Lagrangian
is not Hermitian thus implying a
non-Hermitian Lagrangian 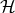 .
.
The quantities to be varied are 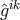 ,
, 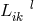 . From
. From 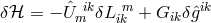 , Einstein then showed
that the first equation of the field equations:
, Einstein then showed
that the first equation of the field equations:
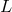 later was named the strong field equations.
later was named the strong field equations.
Equation (200*) replaced the covariant constancy of the metric in general relativity, although, in general,
it does not preserve inner products of vectors propagated parallelly with the same connection. The problem
was already touched in Section 2.1.2: how should we define 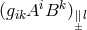 ? M. Pastori has shown that
([485*], p. 112):
? M. Pastori has shown that
([485*], p. 112):
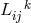 a second one
a second one 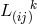 enters.
enters.
7.3 Einstein–Straus 1946 and the weak field equations
It turned out that the result announced, i.e., 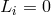 , could not be derived within the formalism
given in the previous paper ([147*]). Together with his assistant Ernst Straus , Einstein wrote a follow-up in which the metric field did not need to be complex [179*].
Equation (199*) now is introduced by a Lagrangian multiplier
, could not be derived within the formalism
given in the previous paper ([147*]). Together with his assistant Ernst Straus , Einstein wrote a follow-up in which the metric field did not need to be complex [179*].
Equation (199*) now is introduced by a Lagrangian multiplier 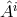 . The new Lagrangian is given by:
. The new Lagrangian is given by:
 is the same quantity as
is the same quantity as 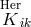 of [147*]; cf. also Eq. (74*) of
Section 2.3.2.95
The variables to be varied independently are
of [147*]; cf. also Eq. (74*) of
Section 2.3.2.95
The variables to be varied independently are 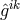 ,
, 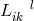 , and the multipliers
, and the multipliers 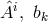 . After some
calculation, the following field equations arose:
The last Eq. (208*) is weaker than
. After some
calculation, the following field equations arose:
The last Eq. (208*) is weaker than ![P [ik] = 0](article984x.gif) ; therefore this system of equations is named the weak field
equations of UFT. However, cf. Section 9.2.2 for a change in Einstein’s wording. From the calculations
involved, it can be seen that (206*) is equivalent to (30*), and that
; therefore this system of equations is named the weak field
equations of UFT. However, cf. Section 9.2.2 for a change in Einstein’s wording. From the calculations
involved, it can be seen that (206*) is equivalent to (30*), and that 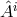 vanishes. The second multiplier
satisfies:
On first sight, according to Eqs. (206*) and (208*), either the skew-symmetric part of
vanishes. The second multiplier
satisfies:
On first sight, according to Eqs. (206*) and (208*), either the skew-symmetric part of 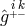 , or the
skew-symmetric part of
, or the
skew-symmetric part of 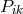 might be related to the electromagnetic field tensor. In the paper, homothetic
curvature
might be related to the electromagnetic field tensor. In the paper, homothetic
curvature 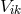 is introduced but not included in the Lagrangian. It will vanish as a consequence of the field
equations given
In linear approximation, the ansatz
is introduced but not included in the Lagrangian. It will vanish as a consequence of the field
equations given
In linear approximation, the ansatz 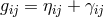 is used with
is used with 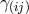 related to the gravitational and
related to the gravitational and
![γ[ij]](article992x.gif) to the electromagnetic field. For the skew-symmetric part
to the electromagnetic field. For the skew-symmetric part ![γ[ij]](article993x.gif) of the metric, the field equations
reduce to
The system (210*), (211*) is weaker than the corresponding Maxwell’s equations in vacuum. According to the
authors, this is no valid objection to the theory “since we do not know to which solutions of the linearized
equations there correspond rigorous solutions which are regular in the entire space.” Only such
solutions are acceptable but: “Whether such (non-trivial) solutions exist is as yet unknown” ([179*],
p. 737).
of the metric, the field equations
reduce to
The system (210*), (211*) is weaker than the corresponding Maxwell’s equations in vacuum. According to the
authors, this is no valid objection to the theory “since we do not know to which solutions of the linearized
equations there correspond rigorous solutions which are regular in the entire space.” Only such
solutions are acceptable but: “Whether such (non-trivial) solutions exist is as yet unknown” ([179*],
p. 737).
Einstein and Straus then discussed whether (207*) and (208*) could be replaced by 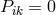 . By
again looking at the linear approximation, they “get a dependence of the electric from the
gravitational field which cannot be brought in accord with our physical knowledge […]” ([179*],
p. 737).
. By
again looking at the linear approximation, they “get a dependence of the electric from the
gravitational field which cannot be brought in accord with our physical knowledge […]” ([179*],
p. 737).
In the long last paragraph of the paper, the authors derive necessary and sufficient (algebraic) conditions for
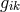 in order that the Eqs. (30*) or (206*) determine the connection (in terms of the metric) “uniquely and without
singularities”. If we set
in order that the Eqs. (30*) or (206*) determine the connection (in terms of the metric) “uniquely and without
singularities”. If we set 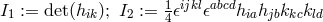 , and
, and 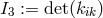 , then they are given
by96:
, then they are given
by96:
Einstein wrote on 6 March 1947 to Schrödinger that he:
“really does not yet know, whether this new system of equations has anything to do with
physics. What justly can be claimed only is that it represents a consequent generalization
of the gravitational equations for empty space.”97
And four months later (16 July 1946), Einstein confessed to Schrödinger:
“As long as the 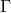 cannot be expressed by the
cannot be expressed by the 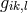 in the simplest way, one cannot hope
to solve exact problems. Due to the diligence and inventiveness of my assistant Straus, we
will have reached this goal, soon.”98
in the simplest way, one cannot hope
to solve exact problems. Due to the diligence and inventiveness of my assistant Straus, we
will have reached this goal, soon.”98
Both quotes are taken from the annotations of K. von Meyenn in ([489*], p. 383). In fall, Pauli who had returned to Zürich wrote to Einstein:
“Schrödinger told me something about you. But I do not know whether you still keep to the field equations which you investigated with Straus at the time of my departure from Princeton (end of February). My personal conviction remains – not the least because of the negative results of your own numerous tries – that classical field theory in whatever form is a completely sucked out lemon from which in no way can spring something new. But I myself do not yet see a path, which leads us further in the principal questions.” ([489*], p. 384)
Unimpressed, Einstein went on squeezing the lemon for the next nine years until his death. On 9 April 1947, he wrote to his friend from student days, Maurice Solovine (1875 – 1958):
“I labour very hard with my Herr Straus at the verification (or falsification) of my
equations. However, we are far from overcoming the mathematical difficulties. It is hard
work for which a true mathematician would not at all muster the courage.” ([160*],
p. 84)99
And, as may be added, for which a genuine true mathematician possibly would not muster enough interest. After all, the task is the resolution of a system of linear equations, well-known in principle, but hard to control for 64 equations. Nevertheless, Einstein’s assistant in Princeton, E. Straus, in dealing with the weak field equations, continued to work at the problem of solving (206*) for the connection. He worked with tensor algebra and presented a formal solution (cf. Eq. (1.9), p. 416 of [592*]). However, it was not only unwieldy but useless in practice. Yet, the mathematical difficulty Einstein blamed for the slow progress made, was “the integration of malicious non-linear equations” (letter to H. Zangger of 28 July 1947 in [560], p. 579).
How appropriate Pauli’s remark was, is made clear by a contemporary paper on “non-symmetric gravity
theories”. Damour, Deser & McCarthy show that the theories of Einstein and Einstein & Straus (together
with further geometrical theories) “violate standard physical requirements” such as to be free of
ghosts100
and with absence of algebraic inconsistencies [101, 102*]. On the other hand, the authors showed that the
following Lagrangian, closely related to an expansion in powers of ![kij = g[ij]](article1006x.gif) of the Einstein–Straus
Lagrangian, would be acceptable:
of the Einstein–Straus
Lagrangian, would be acceptable:
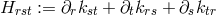 . Indices are moved with the symmetric part of the asymmetric metric
[100].
. Indices are moved with the symmetric part of the asymmetric metric
[100].
Another link from Einstein’s Hermitian theory to modern research leads to “massive gravity” theories, i.e., speculative theories describing an empirically unknown spin-2 particle (graviton) with mass [76, 255]. However, it is not clear whether these theories are free of ghosts.



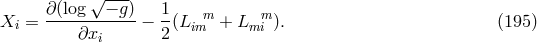
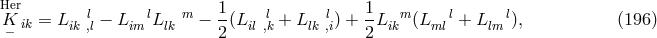

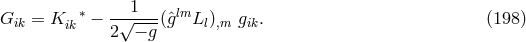

![0 = g ik∥l := gik,l − grkL r− girL r, (200 ) +− il lk Sj (L ) := L [imm] = 0, (201 ) Kjk (L ) = 0 (202 )](article967x.gif)

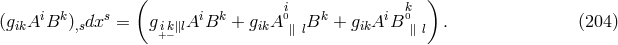
![ℋ = P ˆgik + AˆiL + b ˆg[km] , (205 ) ik i k ,m](article976x.gif)
![Li = 0, ik ˆg+− ||l = 0, (206 ) P(ik) = 0, (207 ) P [ik],l + P[kl],i + P [li],k = 0. (208 )](article983x.gif)
![bi,k − bk,i = 2P [ik]. (209 )](article986x.gif)
![ηjkγ = 0, (210 ) [ij],k ηmn ∂m∂n (γ[ik],l + γ[kl],i + γ[li],k) = 0. (211 )](article994x.gif)

![√ --[ 1 2 ] ℒI = h R (h ) − --HrstHrst − --klm (∂lSm − ∂mSl ) , (213 ) 12 3](article1007x.gif)