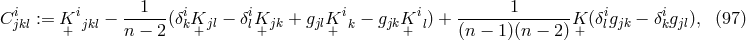2 Mathematical Preliminaries
For the convenience of the reader, some of the mathematical formalism given in the first part of this review is repeated in a slightly extended form: It is complemented by further special material needed for an understanding of papers to be described.
2.1 Metrical structure
First, a definition of the distance 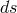 between two infinitesimally close points on a D-dimensional
differential manifold
between two infinitesimally close points on a D-dimensional
differential manifold 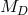 is to be given, eventually corresponding to temporal and spatial distances in the
external world. For
is to be given, eventually corresponding to temporal and spatial distances in the
external world. For 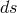 , positivity, symmetry in the two points, and the validity of the triangle equation
are needed. We assume
, positivity, symmetry in the two points, and the validity of the triangle equation
are needed. We assume 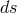 to be homogeneous of degree one in the coordinate differentials
to be homogeneous of degree one in the coordinate differentials
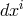 connecting neighboring points. This condition is not very restrictive; it includes Finsler
geometry [510, 199, 394, 4*] to be briefly discussed in Section 17.2.
connecting neighboring points. This condition is not very restrictive; it includes Finsler
geometry [510, 199, 394, 4*] to be briefly discussed in Section 17.2.
In the following, 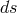 is linked to a non-degenerate bilinear form
is linked to a non-degenerate bilinear form 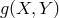 , called the first fundamental
form; the corresponding quadratic form defines a tensor field, the metrical tensor, with
, called the first fundamental
form; the corresponding quadratic form defines a tensor field, the metrical tensor, with 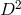 components
components
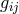 such that
such that
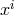 and
and 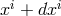 ,
respectively11.
Besides the norm of a vector
,
respectively11.
Besides the norm of a vector 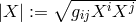 , the “angle” between directions
, the “angle” between directions 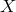 ,
, 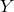 can be defined
by help of the metric:
can be defined
by help of the metric:
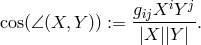
From this we note that an antisymmetric part of the metrical’s tensor does not influence distances and norms but angles.
We are used to 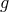 being a symmetric tensor field, i.e., with
being a symmetric tensor field, i.e., with  with only
with only 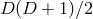 components; in this
case the metric is called Riemannian if its eigenvalues are positive (negative) definite and Lorentzian if its signature is
components; in this
case the metric is called Riemannian if its eigenvalues are positive (negative) definite and Lorentzian if its signature is
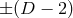 12.
In this case, the norm is
12.
In this case, the norm is 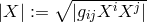 . In space-time, i.e., for
. In space-time, i.e., for 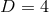 , the Lorentzian signature is
needed for the definition of the light cone:
, the Lorentzian signature is
needed for the definition of the light cone: 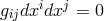 . The paths of light signals through
the cone’s vertex are assumed to lie in this subspace. In unified field theory, the line element
(“metric”)
. The paths of light signals through
the cone’s vertex are assumed to lie in this subspace. In unified field theory, the line element
(“metric”)  is an asymmetric tensor, in general. When of full rank, its inverse
is an asymmetric tensor, in general. When of full rank, its inverse 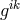 is defined
through13
is defined
through13
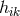 and
and 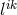 have the same rank; also,
have the same rank; also, 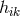 and
and 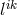 have the same signature [27]. Equation (2*) looks
quite innocuous. When working with the decompositions (3*), (4*) however, eight tensors are floating around:
have the same signature [27]. Equation (2*) looks
quite innocuous. When working with the decompositions (3*), (4*) however, eight tensors are floating around:
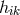 and its inverse
and its inverse 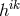 (indices not raised!);
(indices not raised!); 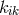 and its inverse
and its inverse 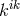 ;
; 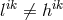 and its inverse
and its inverse
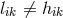 , and finally
, and finally 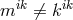 and its inverse
and its inverse 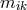 .
.
With the decomposition of the inverse 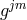 (4*) and the definitions for the respective inverses
(4*) and the definitions for the respective inverses
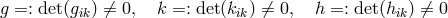 . We also note:
and
Another useful relation is
with
. We also note:
and
Another useful relation is
with 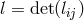 . From (9*) we see that unlike in general relativity even invariants of order zero (in the
derivatives) do exist:
. From (9*) we see that unlike in general relativity even invariants of order zero (in the
derivatives) do exist: 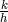 , and
, and 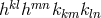 ; for the 24 invariants of the metric of order 1 in space-time
cf. [512, 513, 514].
; for the 24 invariants of the metric of order 1 in space-time
cf. [512, 513, 514].
Another consequence of the asymmetry of 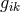 is that the raising and lowering of indices with
is that the raising and lowering of indices with 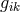 now becomes more complicated. For vector components we must distinguish:
now becomes more complicated. For vector components we must distinguish:
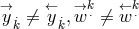 . Fortunately, the raising of indices with the asymmetric metric does not play a role in
the following.
. Fortunately, the raising of indices with the asymmetric metric does not play a role in
the following.
An easier possibility is to raise and lower indices by the symmetric part of 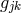 , i.e., by
, i.e., by 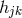 and its inverse
and its inverse
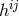 .16
In fact, this is often seen in the literature; cf. [269*, 297*, 298*]. Thus, three new tensors (one symmetric, two
skew) show up:
.16
In fact, this is often seen in the literature; cf. [269*, 297*, 298*]. Thus, three new tensors (one symmetric, two
skew) show up:
![ij ij 1 ij ij ir j. ρ ijrs gg = h[h (1 + 2kijˇk ) + ˇk − ˇk ˇks + 2h-𝜖 krs],](article80x.gif)
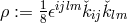 . For a physical theory, the “metric” governing distances and angles must be a
symmetric tensor. There are two obvious simple choices for such a metric in UFT, i.e.,
. For a physical theory, the “metric” governing distances and angles must be a
symmetric tensor. There are two obvious simple choices for such a metric in UFT, i.e., 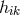 and
and 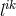 . For
them, in order to be Lorentz metrics,
. For
them, in order to be Lorentz metrics, 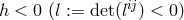 must hold. The light cones
determined by
must hold. The light cones
determined by 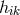 and by
and by 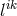 are different, in general. For further choices for the metric
cf. Section 9.7.
are different, in general. For further choices for the metric
cf. Section 9.7.
The tensor density formed from the metric is denoted here by
The components of the flat metric (Minkowski-metric) in Cartesian coordinates is denoted by 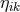 :
:
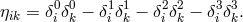
2.1.1 Affine structure
The second structure to be introduced is a linear connection (affine connection, affinity) L with 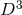 components
components 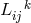 ;
it is a geometrical object but not a tensor field and its components change inhomogeneously under local coordinate
transformations.17
The connection is a device introduced for establishing a comparison of vectors in different points of the
manifold. By its help, a tensorial derivative
;
it is a geometrical object but not a tensor field and its components change inhomogeneously under local coordinate
transformations.17
The connection is a device introduced for establishing a comparison of vectors in different points of the
manifold. By its help, a tensorial derivative  , called covariant derivative is constructed. For each vector
field and each tangent vector it provides another unique vector field. On the components of vector fields X
and linear forms
, called covariant derivative is constructed. For each vector
field and each tangent vector it provides another unique vector field. On the components of vector fields X
and linear forms 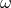 it is defined by
it is defined by
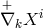 and
and 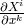 are abbreviated by
are abbreviated by 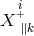 and
and 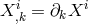 . For a scalar
. For a scalar  ,
covariant and partial derivative coincide:
,
covariant and partial derivative coincide: 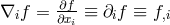 . The antisymmetric part of the
connection, i.e.,
is called torsion; it is a tensor field. The trace of the torsion tensor
. The antisymmetric part of the
connection, i.e.,
is called torsion; it is a tensor field. The trace of the torsion tensor 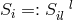 is called torsion vector or
vector torsion; it connects to the two traces of the linear connection
is called torsion vector or
vector torsion; it connects to the two traces of the linear connection 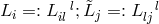 as
as
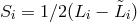 . Torsion is not just one of the many tensor fields to be constructed: it
has a very clear meaning as a deformation of geometry. Two vectors transported parallelly
along each other do not close up to form a parallelogram (cf. Eq. (22*) below). The deficit is
measured by torsion. The rotation
. Torsion is not just one of the many tensor fields to be constructed: it
has a very clear meaning as a deformation of geometry. Two vectors transported parallelly
along each other do not close up to form a parallelogram (cf. Eq. (22*) below). The deficit is
measured by torsion. The rotation 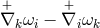 of a 1-form now depends on torsion
of a 1-form now depends on torsion 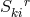 :
:
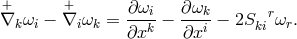
We have adopted the notational convention used by Schouten [537*, 540*, 683]. Eisenhart and others [182*, 438*] change the order of indices of the components of the connection:
whence follows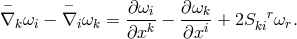
As long as the connection is symmetric this does not make any difference because of
For both kinds of derivatives we have:Both derivatives are used in versions of unified field theory by Einstein and others.18
A manifold provided with only a linear (affine) connection L is called affine space. From the point of
view of group theory, the affine group (linear inhomogeneous coordinate transformations) plays
a special rôle: with regard to it the connection transforms as a tensor; cf. Section 2.1.5 of
Part I.
The covariant derivative with regard to the symmetrical part of the connection 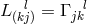 is denoted by
is denoted by 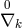 such that19
such that19
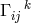 always will denote a symmetric connection if not explicitly defined otherwise. To be
noted is that:
always will denote a symmetric connection if not explicitly defined otherwise. To be
noted is that: ![+ s 0 λ[i,k] = ∇ [kλi] + λsSki = ∇ [kλi]](article126x.gif) .
.
For a vector density of coordinate weight 
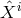 , the covariant derivative contains one more term
(cf. Section 2.1.5 of Part I):
, the covariant derivative contains one more term
(cf. Section 2.1.5 of Part I):
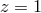 .21
For the concept of gauge weight cf. (491*) of Section 13.2.
.21
For the concept of gauge weight cf. (491*) of Section 13.2.
A smooth vector field Y is said to be parallelly transported along a parametrized curve 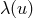 with tangent vector X iff for its components
with tangent vector X iff for its components 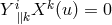 holds along the curve. A
curve is called an autoparallel if its tangent vector is parallelly transported along it in each
point:22
holds along the curve. A
curve is called an autoparallel if its tangent vector is parallelly transported along it in each
point:22
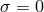 may be reached. Some authors use a
parameter-invariant condition for auto-parallels:
may be reached. Some authors use a
parameter-invariant condition for auto-parallels:  ; cf. [284*].
; cf. [284*].
A transformation mapping auto-parallels to auto-parallels is given by:
The equivalence class of auto-parallels defined by (23*) defines a projective structure on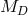 [691], [690].
The particular set of connections
with
[691], [690].
The particular set of connections
with 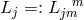 is mapped into itself by the transformation (23*), cf. [608].
is mapped into itself by the transformation (23*), cf. [608].
In Section 2.2.3, we shall find the set of transformations 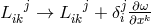 playing a role in versions
of Einstein’s unified field theory.
playing a role in versions
of Einstein’s unified field theory.
From the connection 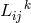 further connections may be constructed by adding an arbitrary tensor field
further connections may be constructed by adding an arbitrary tensor field
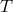 to it or to its symmetrized part:
to it or to its symmetrized part:
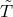 we can regain all connections used in work on unified field theories. One case
is given by Schrödinger’s “star”-connection:
for which
we can regain all connections used in work on unified field theories. One case
is given by Schrödinger’s “star”-connection:
for which 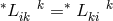 or
or  . The star connection thus shares the vanishing of the torsion vector
with a symmetric connection. Further examples will be encountered in later sections; cf. (382*) of
Section 10.3.3.
. The star connection thus shares the vanishing of the torsion vector
with a symmetric connection. Further examples will be encountered in later sections; cf. (382*) of
Section 10.3.3.
2.1.2 Metric compatibility, non-metricity
We now assume that in affine space also a metric tensor exists. In the case of a symmetric connection the condition for metric compatibility reads:
In Riemannian geometry this condition guaranties that lengths and angles are preserved under parallel transport. The corresponding torsionless connection23 is given by: In place of (28*), for a non-symmetric connection the following equation was introduced by Einstein (and J. M. Thomas) (note the position of the indices!)24 As we have seen in Section 2.1.1, this amounts to the simultaneous use of two connections: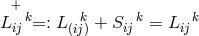 and
and
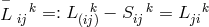 .25
We will name (30*) “compatibility equation” although it has lost its geometrical meaning within Riemannian
geometry.26
In terms of the covariant derivative with regard to the symmetric part of the connection, (30*) reduces to
In the 2nd term on the r.h.s., the upper index has been lowered with the symmetric part
of the metric, i.e., with
.25
We will name (30*) “compatibility equation” although it has lost its geometrical meaning within Riemannian
geometry.26
In terms of the covariant derivative with regard to the symmetric part of the connection, (30*) reduces to
In the 2nd term on the r.h.s., the upper index has been lowered with the symmetric part
of the metric, i.e., with 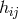 . After splitting the metric into its irreducible parts, we
obtain27
. After splitting the metric into its irreducible parts, we
obtain27
![⋅ r 0 = g i+k−∥l =: hik∥0l + kik∥0l − 2S (i|l|k) + 2kr[iSk]l ,](article156x.gif)
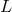 by the metric
and its first partial derivatives. (cf. Section 10.2.3.)
by the metric
and its first partial derivatives. (cf. Section 10.2.3.)
In place of (30*), equivalently, the 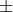 -derivative of the tensor density
-derivative of the tensor density 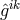 can be made to vanish:
can be made to vanish:
From (30*) or (33*), the connection 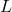 may in principle be determined as a functional of the metric tensor, its first derivatives,
and of torsion.28
After multiplication with
may in principle be determined as a functional of the metric tensor, its first derivatives,
and of torsion.28
After multiplication with 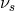 , (33*) can be rewritten as
, (33*) can be rewritten as 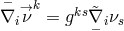 , where
, where
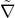 is formed with the Hermitian conjugate connection (cf. Section 2.2.2)
[396].29
is formed with the Hermitian conjugate connection (cf. Section 2.2.2)
[396].29
Remark:
Although used often in research on UFT, the 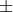 -notation is clumsy and ambiguous. We apply the
-notation is clumsy and ambiguous. We apply the
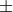 -differentiation to (2*), and obtain:
-differentiation to (2*), and obtain: 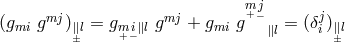 . While the l.h.s. of
the last equation is well defined and must vanish by definition, the r.h.s. is ambiguous and does not vanish:
in both cases
. While the l.h.s. of
the last equation is well defined and must vanish by definition, the r.h.s. is ambiguous and does not vanish:
in both cases 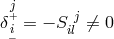 ,
, 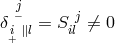 . Einstein had noted this when pointing out that only
. Einstein had noted this when pointing out that only
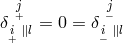 but
but 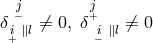 ([147*], p. 580). Already in 1926, J. M. Thomas had seen the
ambiguity of
([147*], p. 580). Already in 1926, J. M. Thomas had seen the
ambiguity of 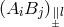 and defined a procedure for keeping valid the product rule for derivatives [607].
Obviously,
and defined a procedure for keeping valid the product rule for derivatives [607].
Obviously, 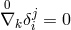 .
.
A clearer presentation of (30*) is given in Koszul-notation:
The l.h.s. of (34*) is the non-metricity tensor, a straightforward generalization from Riemannian geometry: (34*) shows explicitly the occurrence of two connections; it also makes clear the multitude of choices for the non-metricity tensor and metric-compatibility. In principle, Einstein could have also used: and further combinations of the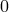 - and
- and 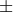 -derivatives. His adoption of (30*) follows from a symmetry
demanded (Hermitian or transposition symmetry); cf. Section 2.2.2.
-derivatives. His adoption of (30*) follows from a symmetry
demanded (Hermitian or transposition symmetry); cf. Section 2.2.2.
An attempt for keeping a property of the covariant derivative in Riemannian geometry, i.e., preservation
of the inner product under parallel transport, has been made by J. Hély [249]. He joined the equations
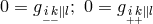 to Eq. (30*). In the presence of a symmetric metric
to Eq. (30*). In the presence of a symmetric metric 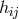 , in place of Eqs. (25*), (26*) a
decomposition
, in place of Eqs. (25*), (26*) a
decomposition
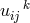 can be made.30
Hély’s additional condition leads to a totally antisymmetric
can be made.30
Hély’s additional condition leads to a totally antisymmetric 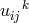 .
.
We will encounter another object and its derivatives, the totally antisymmetric tensor:
where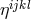 is the totally antisymmetric tensor density containing the entries
is the totally antisymmetric tensor density containing the entries 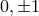 according to whether
two indices are equal, or all indices forming an even or odd permutation. For certain derivatives and
connections, the object can be covariantly constant [473*, 484*]:
according to whether
two indices are equal, or all indices forming an even or odd permutation. For certain derivatives and
connections, the object can be covariantly constant [473*, 484*]:
2.2 Symmetries
2.2.1 Transformation with regard to a Lie group
In Riemannian geometry, a “symmetry” of the metric with regard to a 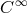 -generator
-generator 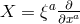 of a
Lie algebra (corresponding, locally, to a Lie-group)
of a
Lie algebra (corresponding, locally, to a Lie-group)
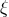 is named a Killing vector; its components generate the infinitesimal symmetry
transformation:
is named a Killing vector; its components generate the infinitesimal symmetry
transformation: 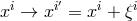 . Equation (43*) may be expressed in a different form:
. Equation (43*) may be expressed in a different form:
In (44*), 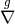 is the covariant derivative with respect to the metric
is the covariant derivative with respect to the metric 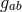 [Levi-Civita connection;
cf. (29*)]. A conformal Killing vector
[Levi-Civita connection;
cf. (29*)]. A conformal Killing vector 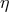 satisfies the equation:
satisfies the equation:
2.2.2 Hermitian symmetry
This is a generalization (a weakening) of the symmetrization of a real symmetric metric and connection:31 Hermitian “conjugate” metric and connection are introduced for a complex metric and connection by
In terms of the real tensors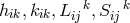 , i.e., of
, i.e., of 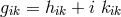 ,
, 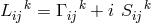 obviously
obviously
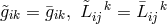 holds, if the symmetry of
holds, if the symmetry of  and the skew-symmetry of
and the skew-symmetry of 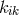 are taken into
account. For a real linear form
are taken into
account. For a real linear form 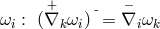 . Hermitian symmetry then means that for both,
metric and connection,
. Hermitian symmetry then means that for both,
metric and connection, 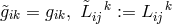 is valid. For the determinant
is valid. For the determinant 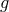 of a metric with
Hermitian symmetry, the relation
of a metric with
Hermitian symmetry, the relation 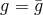 holds.
holds.
The property “Hermitian” (or “self-conjugate”) can be generalized for any pair of adjacent indices of any tensor (cf. [149*], p. 122):
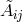 is called the (Hermitian) conjugate tensor. A tensor possesses Hermitian symmetry if
is called the (Hermitian) conjugate tensor. A tensor possesses Hermitian symmetry if
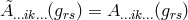 . Einstein calls a tensor anti-Hermitian if
As an example for an anti-Hermitian vector we may take vector torsion
. Einstein calls a tensor anti-Hermitian if
As an example for an anti-Hermitian vector we may take vector torsion ![l Li = L [il]](article221x.gif) with
with 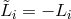 .
The compatibility equation (30*) is Hermitian symmetric; this is the reason why Einstein chose
it.
.
The compatibility equation (30*) is Hermitian symmetric; this is the reason why Einstein chose
it.
For real fields, transposition symmetry replaces Hermitian symmetry.
with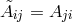 .
.
In place of (47*), M.-A. Tonnelat used
as the definition of a Hermitian quantity [627*]. As an application we find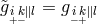 and
and
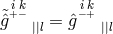 .
.
2.2.3 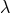 -transformation
-transformation
In (23*) of Section 2.1.1, we noted that transformations of a symmetric connection 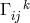 which preserve
auto-parallels are given by:
which preserve
auto-parallels are given by:
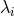 is a real 1-form field. They were named projective by Schouten ([537*], p. 287). In later versions
of his UFT, Einstein introduced a “symmetry”-transformation called
is a real 1-form field. They were named projective by Schouten ([537*], p. 287). In later versions
of his UFT, Einstein introduced a “symmetry”-transformation called 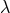 -transformation [156*]:
Einstein named the combination of the “group” of general coordinate transformations and
-transformation [156*]:
Einstein named the combination of the “group” of general coordinate transformations and
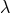 -transformations the “extended” group
-transformations the “extended” group 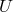 . For an application cf. Section 9.3.1. After gauge-
(Yang–Mills-) theory had become fashionable,
. For an application cf. Section 9.3.1. After gauge-
(Yang–Mills-) theory had become fashionable, 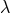 -transformations with
-transformations with 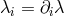 were also interpreted as
gauge-transformations [702*, 23*]. According to him the parts of the connection irreducible with regard to
diffeomorphisms are “mixed” by (52*), apparently because both will then contain the 1-form
were also interpreted as
gauge-transformations [702*, 23*]. According to him the parts of the connection irreducible with regard to
diffeomorphisms are “mixed” by (52*), apparently because both will then contain the 1-form 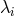 . Under
(52*) the torsion vector transforms like
. Under
(52*) the torsion vector transforms like 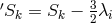 , i.e., it can be made to vanish by a proper choice of
, i.e., it can be made to vanish by a proper choice of
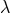 .
.
The compatibility equation (30*) is not conserved under 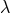 -transformations because of
-transformations because of
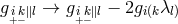 . The same holds for the projective transformations (51*), cf. ([430*], p. 84). No
generally accepted physical interpretation of the
. The same holds for the projective transformations (51*), cf. ([430*], p. 84). No
generally accepted physical interpretation of the 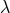 -transformations is known.
-transformations is known.
2.3 Affine geometry
We will speak of affine geometry in particular if only an affine connection exists on the 4-manifold, not a
metric. Thus the concept of curvature is defined.
2.3.1 Curvature
In contrast to Section 2.1.3 of Part I, the two curvature tensors appearing there in Eqs. (I,22) and (I,23)
will now be denoted by the 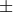 -sign written beneath a letter:
-sign written beneath a letter:
Trivially, for the index pair 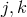 ,
, 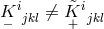 . The curvature tensors (53*), (54*) are skew-symmetric
only in the second pair of indices. A tensor corresponding to the Ricci-tensor in Riemannian geometry is
given by
. The curvature tensors (53*), (54*) are skew-symmetric
only in the second pair of indices. A tensor corresponding to the Ricci-tensor in Riemannian geometry is
given by
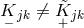 when
when 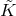 denotes the Hermitian (transposition) conjugate. Thus, in
general
denotes the Hermitian (transposition) conjugate. Thus, in
general
If the curvature tensor for the symmetric part of the connection is introduced by:
then The corresponding expression for the Ricci-tensor is: whence follows: with . Also, the relations hold (for (63*) cf. [549*], Eq. (2,12), p. 278)):
A consequence of (62*), (63*) is:
Another trace of the curvature tensor exists, the so-called homothetic
curvature32:
Likewise,
such that
. Also, the relations hold (for (63*) cf. [549*], Eq. (2,12), p. 278)):
A consequence of (62*), (63*) is:
Another trace of the curvature tensor exists, the so-called homothetic
curvature32:
Likewise,
such that 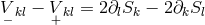 . For the curvature tensor, the identities hold:
where the bracket
. For the curvature tensor, the identities hold:
where the bracket 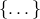 denotes cyclic permutation while the index
denotes cyclic permutation while the index 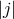 does not take part.
does not take part.
Equation (68*) generalizes Bianchi’s identity. Contraction on 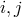 leads to:
leads to:
![V + 2K = 0. jk [jk]](article269x.gif)
Finally, two curvature scalars can be formed:
For a symmetric connection, an additional identity named after O. Veblen holds:
The integrability condition for (30*) is ([399*], p. 225), [51]:
For a complete decomposition of the curvature tensor (53*) into irreducible parts with regard to the
permutation group further objects are needed, as e.g., ![ajkl b ajkl b 𝜖 K+ jkl = 2𝜖 ∂[kSl]j](article273x.gif) ; cf. [348].
; cf. [348].
2.3.2 A list of “Ricci”-tensors
In many approaches to the field equations of UFT, a generalization of the Ricci scalar serves as a Lagrangian.
Thus, the choice of the appropriate “Ricci” tensor plays a distinct role. As exemplified by Eq. (64*), besides
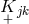 and
and 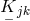 there exist many possibilities for building 2-rank tensors which could form a substitute for
the unique Ricci-tensor of Riemannian geometry. In ([150*], p. 142), Einstein gives a list of 4 tensors
following from a “single contraction of the curvature tensor”. Santalò derived an 8-parameter set of
“Ricci”-type tensors constructed by help of
there exist many possibilities for building 2-rank tensors which could form a substitute for
the unique Ricci-tensor of Riemannian geometry. In ([150*], p. 142), Einstein gives a list of 4 tensors
following from a “single contraction of the curvature tensor”. Santalò derived an 8-parameter set of
“Ricci”-type tensors constructed by help of 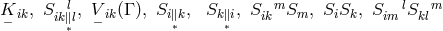 ([524*], p. 345). He discusses seven of them used by Einstein, Tonnelat, and
Winogradzki.33
The following collection contains a few examples of the objects used as a Ricci-tensor in
variational principles/field equations of UFT besides
([524*], p. 345). He discusses seven of them used by Einstein, Tonnelat, and
Winogradzki.33
The following collection contains a few examples of the objects used as a Ricci-tensor in
variational principles/field equations of UFT besides 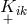 and
and 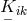 of the previous
section.34
They all differ in terms built from torsion. Among them are:
of the previous
section.34
They all differ in terms built from torsion. Among them are:
One of the puzzles remaining in Einstein’s research on UFT is his optimism in the search for a preferred Ricci-tensor although he had known, already in 1931, that presence of torsion makes the problem ambiguous, at best. At that time, he had found a totality of four possible field equations within his teleparallelism theory [176]. As the preceding list shows, now a 6-parameter object could be formed. The additional symmetries without physical support suggested by Einstein did not help. Possibly, he was too much influenced by the quasi-uniqueness of his field equations for the gravitational field.
2.3.3 Curvature and scalar densities
From the expressions (73*) to (81*) we can form scalar densities of the type: 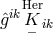 to
to 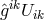 etc. As the
preceding formulas show, it would be sufficient to just pick
etc. As the
preceding formulas show, it would be sufficient to just pick 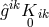 and add scalar densities built from
homothetic curvature, torsion and its first derivatives in order to form a most general Lagrangian. As will
be discussed in Section 19.1.1, this would draw criticism to the extent that such a theory does not qualify
as a unified field theory in a stronger sense.
and add scalar densities built from
homothetic curvature, torsion and its first derivatives in order to form a most general Lagrangian. As will
be discussed in Section 19.1.1, this would draw criticism to the extent that such a theory does not qualify
as a unified field theory in a stronger sense.
2.3.4 Curvature and  -transformation
-transformation
The effect of a 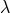 -transformation (52*) on the curvature tensor
-transformation (52*) on the curvature tensor  is:
is:
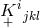 is used, instead of (52*) we must take the form for the
is used, instead of (52*) we must take the form for the
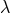 -transformation:35
-transformation:35
Then
also holds. Application of (52*) to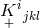 , or (85*) to
, or (85*) to 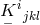 results in many more terms in
results in many more terms in
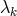 on the r.h.s. For the contracted curvatures a
on the r.h.s. For the contracted curvatures a 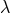 -transformation leads to (cf. also [430]):
If
-transformation leads to (cf. also [430]):
If 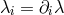 , the curvature tensors and their traces are invariant with regard to the
, the curvature tensors and their traces are invariant with regard to the 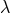 -transformations of
Eq. (52*). Occasionally,
-transformations of
Eq. (52*). Occasionally, 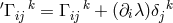 is interpreted as a gravitational gauge transformation.
is interpreted as a gravitational gauge transformation.
2.4 Differential forms
In this section, we repeat and slightly extend the material of Section 2.1.4, Part I, concerning Cartan’s
one-form formalism in order to make understandable part of the literature. Cartan introduced one-forms
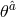 (
(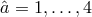 ) by
) by 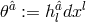 . The reciprocal basis in tangent space is given by
. The reciprocal basis in tangent space is given by 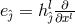 .
Thus,
.
Thus, 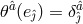 . An antisymmetric, distributive and associative product, the external or
“wedge”(
. An antisymmetric, distributive and associative product, the external or
“wedge”(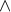 )-product is defined for differential forms. Likewise, an external derivative
)-product is defined for differential forms. Likewise, an external derivative 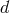 can be
introduced.36
The metric (e.g., of space-time) is given by
can be
introduced.36
The metric (e.g., of space-time) is given by 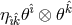 , or
, or 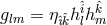 . The covariant derivative of a
tangent vector with bein-components
. The covariant derivative of a
tangent vector with bein-components 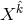 is defined via Cartan’s first structure equations,
is defined via Cartan’s first structure equations,
 is the connection-1-form, and
is the connection-1-form, and 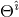 is the torsion-2-form,
is the torsion-2-form, 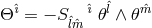 .
We have
.
We have  . The link to the components
. The link to the components ![L[ijk]](article325x.gif) of the affine connection is given by
of the affine connection is given by
 37.
The covariant derivative of a tangent vector with bein-components
37.
The covariant derivative of a tangent vector with bein-components 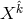 then is
then is
By further external derivation on 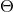 we arrive at the second structure relation of Cartan,
we arrive at the second structure relation of Cartan,
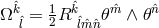 appears, which is given by
appears, which is given by
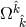 is the homothetic curvature.
is the homothetic curvature.
A p-form in n-dimensional space is defined by

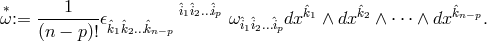
2.5 Classification of geometries
A differentiable manifold with an affine structure is called affine geometry. If both, a (possibly
non-symmetric) “metric” and an affine structure, are present we name the geometry “mixed”. A subcase,
i.e., metric-affine geometry demands for a symmetric metric. When interpreted just as a gravitational
theory, it sometimes is called MAG. A further subdivision derives from the non-metricity tensor being zero
or 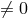 . Riemann–Cartan geometry is the special case of metric-affine geometry with vanishing
non-metricity tensor and non-vanishing torsion. Weyl’s geometry had non-vanishing non-metricity tensor
but vanishing torsion. In Sections 2.1.3 and 4.1.1 of Part I, these geometries were described in greater
detail.
. Riemann–Cartan geometry is the special case of metric-affine geometry with vanishing
non-metricity tensor and non-vanishing torsion. Weyl’s geometry had non-vanishing non-metricity tensor
but vanishing torsion. In Sections 2.1.3 and 4.1.1 of Part I, these geometries were described in greater
detail.
2.5.1 Generalized Riemann-Cartan geometry
For the geometrization of the long-range fields, various geometric frameworks have been chosen. Spaces with a connection depending solely on a metric as in Riemannian geometry rarely have been considered in UFT. One example is given by Hattori’s connection, in which both the symmetric and the skew part of the asymmetric metric enter the connection39 [240*]:
where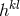 is the inverse of
is the inverse of 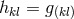 . As described in Section 6.2 of Part I, its physical content is
dubious. As the torsion tensor does not vanish, in general, i.e.,
this geometry could be classified as generalized Riemann–Cartan geometry.
. As described in Section 6.2 of Part I, its physical content is
dubious. As the torsion tensor does not vanish, in general, i.e.,
this geometry could be classified as generalized Riemann–Cartan geometry.
2.5.2 Mixed geometry
Now, further scalars and scalar densities may be constructed, among them curvature scalars (Ricci-scalars):
Here,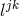 and
and 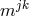 come from the decomposition into irreducible parts of the inverse of the
non-symmetric metric
come from the decomposition into irreducible parts of the inverse of the
non-symmetric metric 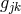 . Both parts on the r.h.s. could be taken as a Lagrangian, separately. The
inverse
. Both parts on the r.h.s. could be taken as a Lagrangian, separately. The
inverse  of the symmetric part, i.e., of
of the symmetric part, i.e., of 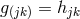 could be used as well to build a scalar:
could be used as well to build a scalar: 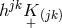 .
Mixed geometry is the one richest in geometrical objects to be constructed from the asymmetric metric and
the asymmetric connection. What at first may have appeared as an advantage, turned out to become an
‘embarras de richesses’: defining relations among geometric objects and physical observables abound;
cf. Section 9.7.
.
Mixed geometry is the one richest in geometrical objects to be constructed from the asymmetric metric and
the asymmetric connection. What at first may have appeared as an advantage, turned out to become an
‘embarras de richesses’: defining relations among geometric objects and physical observables abound;
cf. Section 9.7.
Whenever a symmetric tensor appears which is independent of the connection and of full rank, it can play the role of a metric. The geometry then may be considered to be a Riemannian geometry with additional geometric objects: torsion tensor, non-metricity tensor, skew-symmetric part of the “metric” etc. These might be related to physical observables. Therefore, it is moot to believe that two theories are different solely on the basis of the criterion that they can be interpreted either in a background of Riemannian or mixed geometry. However, by a reduction of the more general geometries to a mere Riemannian one plus some additional geometric objects the very spirit of UFT as understood by Einstein would become deformed; UFT explicitly looks for fundamental geometric objects representing the various physical fields to be described.
2.5.3 Conformal geometry
This is an “angle preserving” geometry: in place of a metric  a whole equivalence class
a whole equivalence class
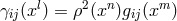 with a function
with a function  obtains. Geometrical objects of interest are those
invariant with regard to the transformation:
obtains. Geometrical objects of interest are those
invariant with regard to the transformation: 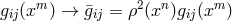 . One such object is Weyl’s
conformal curvature tensor:
. One such object is Weyl’s
conformal curvature tensor:
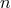 is the dimension of the manifold (
is the dimension of the manifold (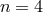 : space-time).
: space-time). 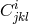 is trace-free. For
is trace-free. For 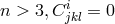 is a necessary and sufficient condition that the space is conformally flat, i.e.,
is a necessary and sufficient condition that the space is conformally flat, i.e., 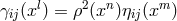 ([191], p. 92).
([191], p. 92).
If 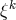 is a Killing vector field for
is a Killing vector field for 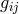 , then
, then 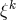 is a conformal Killing vector field for
is a conformal Killing vector field for 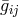 ;
cf. Eq. (45*) in Section 2.1.2.
;
cf. Eq. (45*) in Section 2.1.2.
A particular sub-case of conformal geometry is “similarity geometry”, for which the restricted group of
transformations acts 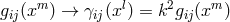 , with a constant
, with a constant 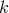 , cf. Section 3.1.
, cf. Section 3.1.
2.6 Number fields
In Section 2.3 of Part I, the possibility of choosing number fields different from the real numbers for the field variables was stated. Such field variables then would act in a manifold with real coordinates. A more deeply going change is the move to an underlying manifold with coordinates taken from another number field, e.g., complex spaces. The complex number field was most often used in connection with unified field theory in both roles. cf. A. Einstein, (complex space, Section 7.2), J. Moffat, (complex field on real space, Section 13) and A. Crumeyrolle, (hypercomplex manifold, Section 11.2.2).
As hypercomplex numbers are less well known, we briefly introduce them here. Let 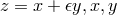 real and consider the algebra with two elements
real and consider the algebra with two elements 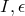 , where
, where 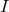 is the unit element and
is the unit element and 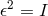 .
.  is
called a hypercomplex number. A function
is
called a hypercomplex number. A function 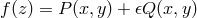 will be differentiable in
will be differentiable in  if
if




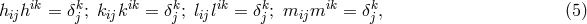
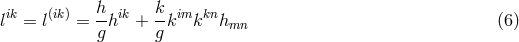
![ik [ik] k ik h im kn m = m = -k + -h h kmn (7 ) g g](article62x.gif)

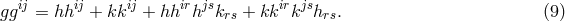



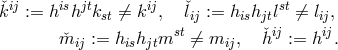
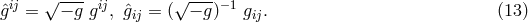
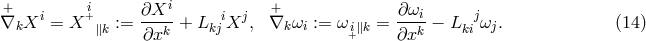
![Sij k= L[ijk] (15 )](article101x.gif)
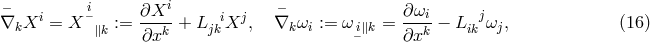
![+ i − i i j ∇kX − ∇kX = 2S[kj] X = 0. (17 )](article110x.gif)
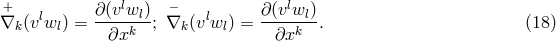
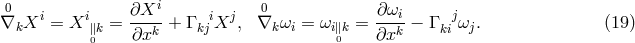
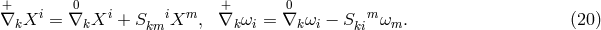
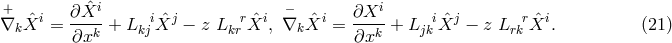



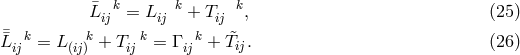

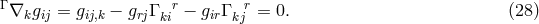
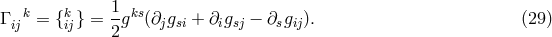
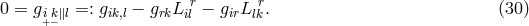
![⋅ r 0 = g+ik−∥l =: gik∥0l − 2S (i|l|k) + 2kr[iSk]l . (31 )](article154x.gif)
![r r hik∥0l + 2hr (iSk)l = 0, kik∥0l + 2kr[iSk]l = 0. (32 )](article157x.gif)
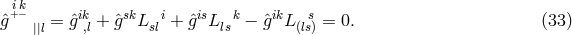
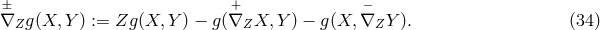
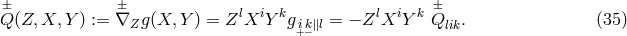
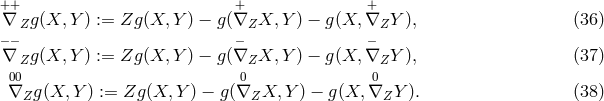

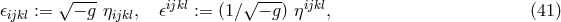
![ijk l ijkl 𝜖 = 0, 𝜖++++ = 𝜖−−−− = 𝜖ijklL s. (42 ) i0j0k00l||r ||r ||r [rs]](article194x.gif)
![l [X (i),X (j)] = cij X (l),](article197x.gif)
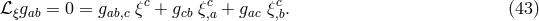




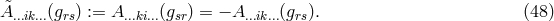
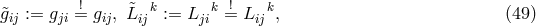



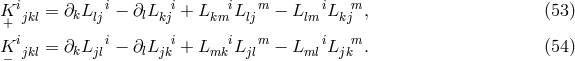
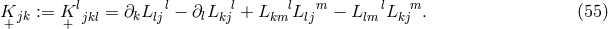
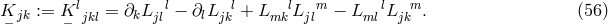
![− K [jk] := ∂[kSj] + ∇lSlkj. (57 ) −](article252x.gif)

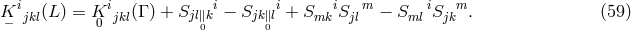
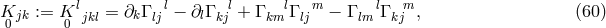
![K0 [jk] := ∂[kΓ j] (61 )](article256x.gif)
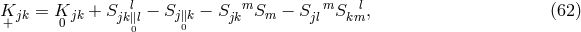
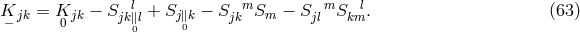
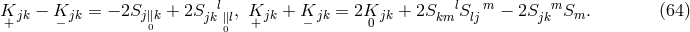


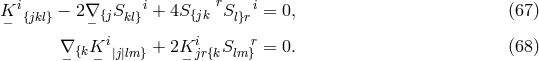
![V + 2K = 2∇ S l+ 4S rS + 4∇ S , (69 ) jk [jk] l jk jk r [j k]](article268x.gif)

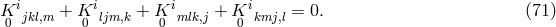

![Her 1 K− ik = − 2-(K− ik + &tidle;K− ik) = Pik = L ik l,l − LimlL lk m − 1-(Lill ,k + L lk l,i) + 1L ikm (L mll+ Llml)([147 ], p. 581 ) (73 ) 2 2 1- &tidle; l l m = 2(K0 ik + K0 ik) + Sik∥0l + Sim Skl ; (74 ) 1 1 P ∗ik = L ik l,l − Lim lL lk m − -(L(il)l ,k + L(lkl) ,i) +-Likm(L mll+ L lm l)([150], p. 142) (75 ) 2 2 Her = K− ik + S[i,k]([371], p. 247–248) (76 ) 2 (1)Rik = − K ik + --(∂iSk − ∂kSi )([632], p. 129); (77 ) − 3 (2)R = ∂ L l− ∂ L l+ L lL m − L lL m + 1(∂ S − ∂ S ) − 1S S ([632], p. 129) ik l ik k (il) ik (lm) im lk 3 i k k i 3 i k 2 l m 1 m 1 = − K0 ik − 3-S[i∥k] + Sim S kl + 3S ik Sm − 3SiSk; (78 ) 0 (3)Rik = (2)Rik − 1V ik,([632], p. 129); (79 ) 2+ Her 1 Uik = K− ik − --[Si,k − Sk,i + SiSk] (80 ) 3 = K ik − Sikl∥l + Sim lS kml − 2S [i∥k] − 2S ikm Sm − 1SiSk,([151], p. 137; (81 ) 0 0 3 0 3 3 ∗ + l l m l 1 m l l R ik = − K ik + ∇kSi = L ik ,l − L im L lk − L (il) ,k +--Lik (L ml + L lm )([156], p. 144) (82 ) − ∗∗ 2∗ √ --- R ik = R ik − [(log( − g)),i]||k([156], p. 144) (83 ) −](article284x.gif)
![K i → K i + 2 ∂ λ δ i. (84 ) − jkl − jkl [k l] j](article291x.gif)

![K ijkl → K ijkl + 2 ∂[kλl]δji. (86 ) + +](article296x.gif)
![K jk → K jk − 2∂[kλj], V jk → V jk + 2∂[jλk], V jk → V jk + 8∂ [jλk]. (87 ) − − + + − −](article301x.gif)




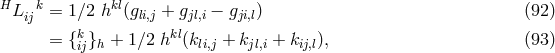
![H k kl Sij = h (kl[i,j] + 1∕2kij,l) (94 )](article348x.gif)
![jk jk jk K+ := g K+jk = l K+ (jk) + m K+ [jk], (95 ) jk jk jk K− := g K− jk := l K− (jk) + m K− [jk]. (96 )](article349x.gif)